monometey & buoyemcy

 use the relationship between pressure and head to measure pressure. 

Manometers: Manometers are used for measuring pressures by balancing the fluid column of fluid against another column of fluid of known specific gravity.

• Pressure is proportional to the height of a column of fluid.
• The instrument used to carry out the complete process is termed as Manometer.
• Types of Manometers: Barometer, Piezometer and U-tube Manometer.
• Manometers use the relationship between pressure and head to measure pressure 

       Piezometer

• The simplest manometer is an open tube. This is attached to the top of a container with liquid at pressure. containing liquid at a pressure.
• The tube is open to the atmosphere, the pressure measured is relative to atmospheric so it measures gauge pressure.

                                                                                                                          Fig. Piezometer

 

 P = ρgH

Where,

ρ = density of liquid

h = height of liquid in the piezometer from the centre of the pipe.

g = acceleration due to gravity.

Limitations of Piezometer -

• Can only be used for liquids.
• Pressure must above atmospheric.
• Liquid height must be convenient i.e. not be too small or too large.

U-tube Manometer

• It consist a U shaped bend whose one end is attached to the gauge point ‘A’ and the other end is open to the atmosphere.
• It can measure both positive and negative (suction) pressures.
• U-Tube enables the pressure of both liquids and gases to be measured U is connected as shown and filled with manometric fluid.

Note.

• The manometric fluid density should be greater than of the fluid measured, ρ_mano. > ρ
• The two fluids should not be able to mix they must be immiscible.

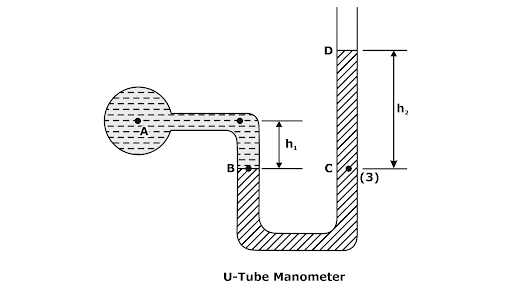
  Fig. U-Tube Manometer

• Pressure in a continuous static fluid is the same at any horizontal level,

        Pressure at B = Pressure at C

                             P_B = P_C

• For the left-hand arm pressure at B,

      P_B = Pressure at A + Pressure of height of liquid being measured

      P_B = P_A + ρgh₁

• For the right-hand arm pressure at C,
• P_C  = Pressure at D + Pressure of height of manometric liquid, 

      P_C =  ρ_mano gh2

We are measuring gauge pressure we can subtract P_atmospheric giving

       P_B = P_C

       P_A  = ρ_manogh2 – ρgh₁

Differential U-Tube Manometer,

• A U-Tube manometric liquid is heavier than the liquid for which the pressure difference is to be measured and is not immiscible with it.

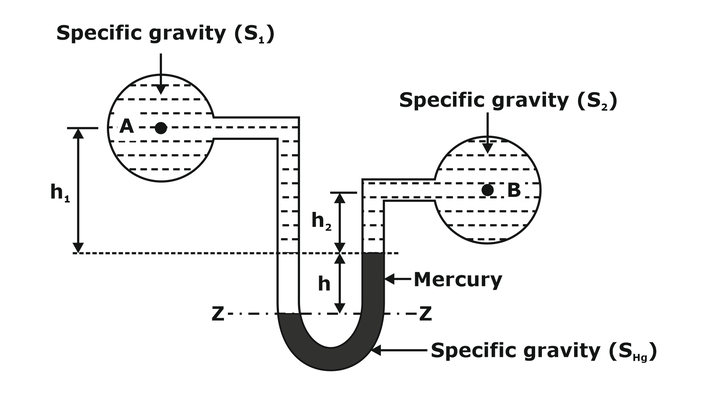
                Fig. Differential U-tube manometer

    Pressure difference between A and B is given by equation

Inverted U-Tube Manometer:

• An inverted U-tube manometer consists of an inverted U-tube containing a light liquid.
• This is used to measure the differences of low pressures between two points where better accuracy is required.

Fig. Inverted U-Tube Manometer

Pressure difference can be calculated from equation,

Micro Manometer

• Micro Manometer is the modified form of a simple manometer whose one limb is made of larger cross-sectional area.
• It measures very small pressure differences with high precision.

Inclined Manometer

• An inclined manometer is used for the measurement of small pressures and is to measure more accurately than the vertical tube type manometer.
• Due to inclination, the distance moved by the fluid in the manometer is more.

 

 

 Buoyancy

Buoyancy is also known as the buoyant force. It is the force exerted on an object that is wholly or partly immersed in a fluid.

Archimedes Principle:

• Whenever a body is immersed in fluid either partially on fully, it experiences a net vertical force which is known as buoyant force.
• This buoyant force is equal to the weight of the fluid displaced by the body, and it acts upward through the centroid of the displaced volume.

Concept of Buoyancy: 

• The point of application of buoyancy force is called the center of buoyancy and it coincides with the centroid of the wholly submerged body of the homogenous composition.
• This buoyant force is equal to the weight of the fluid displaced by the body, and it acts upward through the centroid of the displaced volume.

• For floating bodies, the weight of the entire body must be equal to the buoyant force, which is the weight of the fluid whose volume is equal to the volume of the submerged portion of the floating body i.e.

 Where,

F_B=Buoyancy force,

V_disp.= Displacement volume

Vbody= Total volume of the body,

ρ_l = Density of liquid

ρ_b = Density of body,

 Stability conditions of completely submerged bodies under angular deflection:

The rotational stability of an immersed body depends on the relative locations of the center of gravity (G) of the body and the center of buoyancy(B).

(a). For the stable equilibrium: B should be above G.

(b). For the unstable equilibrium: B should be below G.

(c). For the neutral equilibrium: B and G coincide.

Fig. Illustration of the stability conditions of the completely submerged bodies.

 

Metacentric height (GM):

The metacentric height GM, which is the distance between the center of gravity (G) and the metacenter (M) which is the intersection point of the lines of action of the buoyant force through the body before and after rotation.

The metacentric height using the theoretical method is given by the following formula,

In the above expression,

BM= Metacentric height,

BG=Calculated from geometry.

• Positive (+Ve) sign is used when the metacenter M lies above the G.
• Negative (-Ve) sign is used when the metacenter M lies below the G.

 

 Stability conditions of partially submerged bodies under angular deflection:

The rotational stability of an immersed body depends on the relative locations of the Metacenter (M) of the body and the center of gravity (G).

For a floating body to be in:

(a). stable equilibrium, point M should be above point G and thus GM is positive. The larger the GM is, the more stable is the floating body.

(b). unstable equilibrium, point M should be below point G and thus GM is negative.

(c). neutral equilibrium, point M should coincide with the G and thus GM =0.

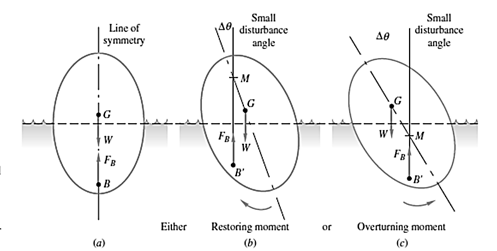Fig. Illustration of stability conditions of the Floating bodies

 

 Rolling and Pitching:

The angular displacement of a boat or ship about its longitudinal axis is known as rolling while that about its transverse axis is known as pitching.

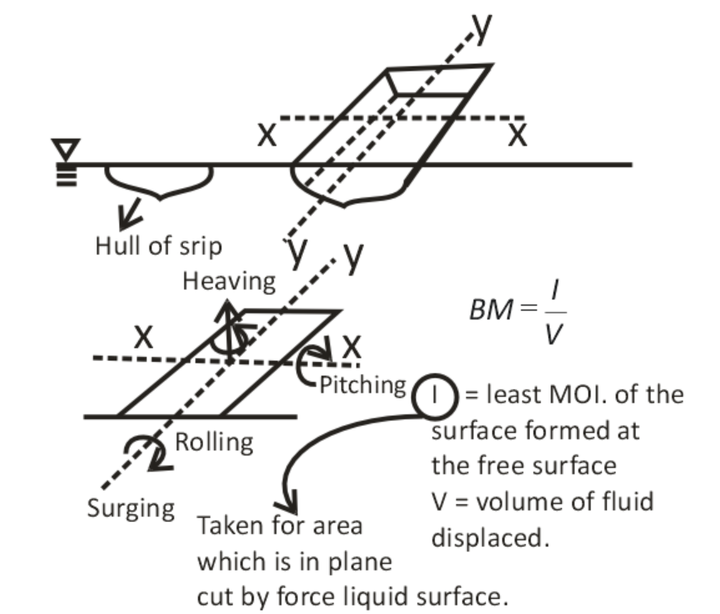
 Fig. Illustration of the rolling and pitching of a hull

 

Time period of Oscillation of the floating body,

 The time period of the oscillation is given by the following expression,

Increasing the metacentric height gives greater stability but reduces the time period of the roll so the ship will be less comfortable for the passengers.