dimensional parameters in free & forced convection

The following dimensionless parameters are significant in evaluating the convection heat transfer coefficient:

• It is a dimensionless quantity defined as: hL/ k,
  where h = convective heat transfer coefficient
  L is the characteristic length 
  k is the thermal conductivity of the fluid.

• The Nusselt number could be interpreted physically as the ratio of the temperature gradient in the fluid immediately in contact with the surface to a reference temperature gradient (T_s - T_∞) /L.
• The convective heat transfer coefficient can easily be obtained if the Nusselt number, the thermal conductivity of the fluid in that temperature range and the characteristic dimension of the object is known.
• Let us consider a hot flat plate (temperature T_w) placed in a free stream (temperature T_∞ < T_w). The temperature distribution is shown in the following figure.

• Newton's Law of Cooling says that the rate of heat transfer per unit area by convection is given by:
  
  
  
  

• Nu measure energy transfer by convection occurring at the surface. Larger the value of Nu, larger will be the rate of heat transfer by convection.

Temperature distribution in a boundary layer: Nusselt modulus

• The heat transfer by convection involves conduction and mixing motion of fluid particles. At the solid fluid interface (y = 0), the heat flows by conduction only, and is given by:
  

• Since the magnitude of the temperature gradient in the fluid will remain the same, irrespective of the reference temperature, we can write dT = d(T - Tw) and by introducing a characteristic length dimension L to indicate the geometry of the object from which the heat flows:
  
  

In dimensionless form:

Reynold Number (Re):

• Reynold number is defined as:
  
  
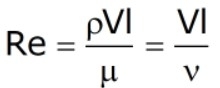
  where: ρ = Density of fluid
  V = Velocity of fluid passing through length (l)
  ν = Kinematic viscosity
  μ = Dynamic viscosity 

Critical Reynold Number: It represents the number where the boundary layer changes from laminar to turbine flow.

(a). For flat plate

• Re < 5 × 10⁵ (laminar)
• Re > 5 × 10⁵ (turbulent)

(b). For circular pipes

• Re < 2300 (laminar flow)
• 2300 < Re < 4000 (transition to turbulent flow)
• Re > 4000 (turbulent flow)

Stanton Number (St): 

It is the measure of the ratio of heat transferred to the fluid and its thermal capacity.

Grashof Number (Gr)

• In natural or free convection heat transfer, die motion of fluid particles is created due to buoyancy effects. The driving force for fluid motion is the body force arising from the temperature gradient.
• If a body with a constant wall temperature T_w is exposed to a quiescent ambient fluid at T_∞, the force per unit volume can be written as:
  ρgβ(t_w - t_∞)
  where ρ: density of the fluid
  β: volume coefficient of expansion
  g: acceleration due to gravity.

• It is used for free convection.
  
  where, β = Coefficient of volumetric expansion = 1/T

• The ratio of inertia force × Buoyancy force/(viscous force)² can be written as

• The magnitude of Grashof number indicates whether the flow is laminar or turbulent.
• If the Grashof number is greater than 10⁹, the flow is turbulent and
• If Grashof number less than 10⁸, the flow is laminar.
• For 10⁸ < Gr < 10⁹, It is the transition range.

Prandtl Number (Pr)

• It is a dimensionless parameter defined as:
  
  
  
  where μ: dynamic viscosity of the fluid
  v = kinematic viscosity
  α = thermal diffusivity

• For liquid metal: Pr < 0.01
• For air and gases: Pr ≈1
• For water: Pr ≈10
• For heavy oil and grease: Pr > 10⁵
• It provides a measure of relative effective of momentum and energy transport by diffusion in velocity and thermal boundary layers respectively. Higher Pr means higher Nu and it shows higher heat transfer.
• This number assumes significance when both momentum and energy are propagated through the system. It is a physical parameter depending upon the properties of the medium.
• It is a measure of the relative magnitudes of momentum and thermal diffusion in the fluid:
  For Pr = 1, Thermal boundary layer thickness (δ_t) = momentum boundary layer (δ)
  For Pr << 1 (Liquid metals), δ_t >>δ
• The product of Grashof and Prandtl number is called Rayleigh number.
  Ra = Gr × Pr.

Rayleigh Number (Ra):

• It is the product of Grashof number and Prandtl number. It is used for free convection.
  where:
  g: Acceleration due to gravity
  β: Thermal expansion coefficient
  v: Kinematic viscosity
  α: Thermal diffusivity
  Pr: Prandtl number
  Gr: Grashof number
• In free or natural convection:
  For laminar flow: 10⁴ < Ra < 10⁹ 
  For turbulent flow: Ra > 10⁹.