Elastic constants
Elastic constants are those factors which determine the deformations produced by a given stress system acting on a material.
Various elastic constants are :
(i) Modulus of elasticity (E)
(ii) Poisson’s ratio (μ or 1/m)
(iii) Modulus of rigidity (G or N)
(iv) Bulk modulus (K)
Materials on the basis of elastic properties
(i)Homogeneous Material
When a material exhibits Same elastic properties at any point in a given directions than the material is known as homogenous material.
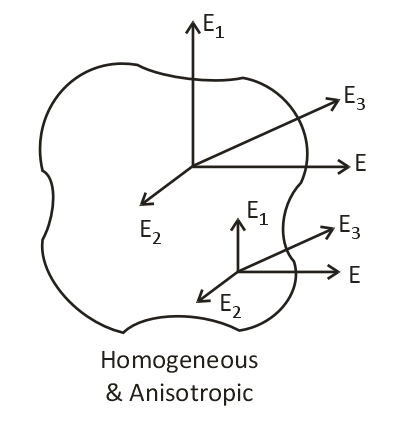
Fig. Homogeneous material
(ii)Isotropic Material
When a material exhibits Same elastic properties at any direction at a given point than the material is known as Isotropic Material.
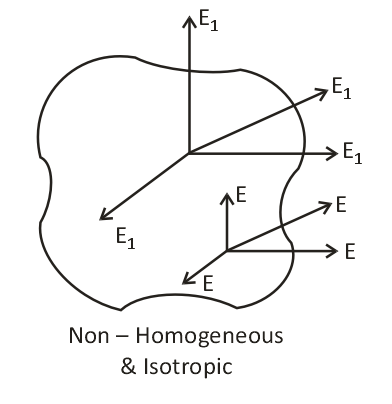
Fig. Non-Homogenous and isotropic material
(iii)Anisotropic Material
When a material exhibits different elastic properties at every direction at a every point than the material is known as Isotropic Material.
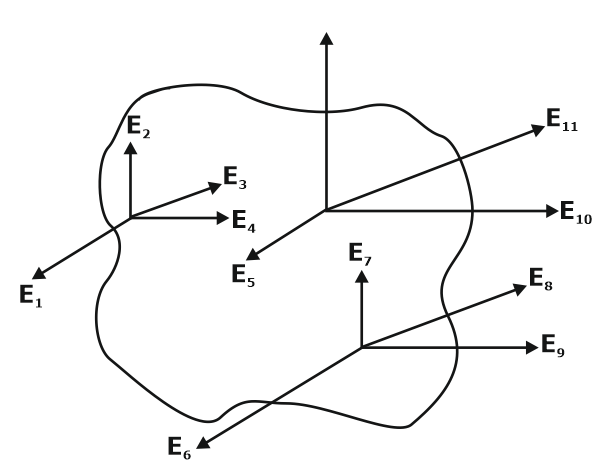
Fig. Anisotropic material
(v) Orthotropic Material
When a material exhibits Same elastic properties at only orthogonal direction at a given point than the material is known as Orthotropic Material.
For a homogeneous and isotropic material, the number of independent elastic constants are two.
Material | No. of independent elastic constants |
Isotropic | 2 |
Orthotropic | 9 |
Anisotropic | 21 |
MODULUS OF ELASTICITY
When an axial load, P is applied along the longitudinal axis of a bar due to which length of the bar will be increases in the direction of applied load and a stress, σ is induced in the bar.
The ratio of stress to longitudinal strain, within elastic limits, is called the modulus of elasticity (E):
![]()
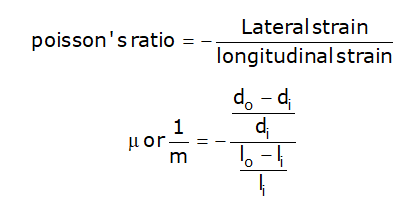
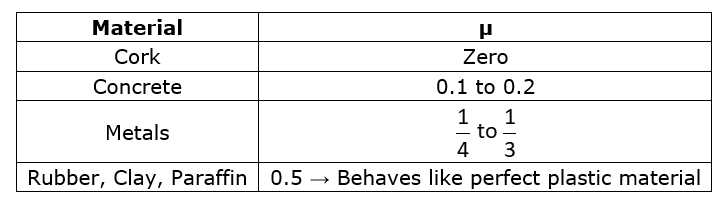
POISSON’S RATIO
It is the ratio of lateral strain to the longitudinal strain.
It is an unitless quantity which is generally denoted as μ or 1/m.
VOLUMETRIC STRAIN
Volumetric Strain Due to Three Mutually Perpendicular Stress
Figure shows a parallelepiped subjected to three tensile load P1, P2 and P3 in the three mutually perpendicular direction.
Then,
![]()
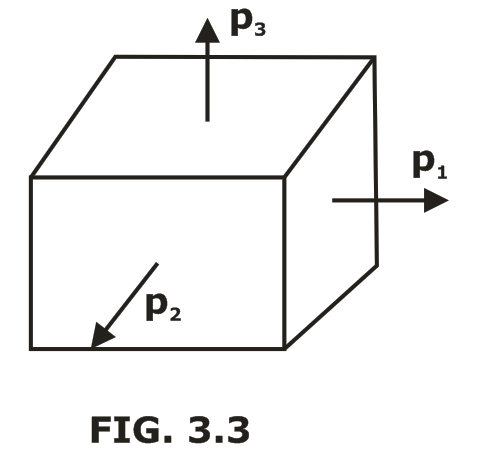
Fig. Parallelepiped subjected to Three Mutually Perpendicular Stress
Since any axial load produces a strain in its own direction and an opposite kind of strain in every direction at right angles to this direction.
we have,
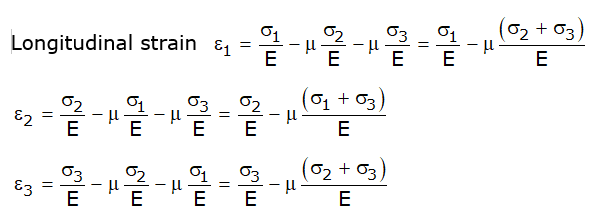
Adding the three expressions of Equations we get.
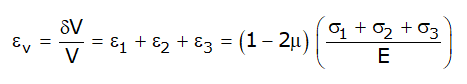
Hydrostatic static state of stress-
In case of hydrostatic state of stress, the applied stress in all direction are equal and tensile in nature.
i.e. σ1 = σ2 = σ3 = σ
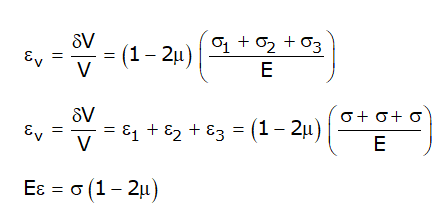
since Eϵ and σ in the above expression are positive numbers, must also be positive.

Thus, maximum value poison’s ratio is 0.5
Volumetric Strain Due to Single Direct Stress
Figure shows a rectangular bar of length L, width b and thickness t subjected to single direct load (P) acting along its longitudinal axis. Let this stress σ generated to be tensile in nature.
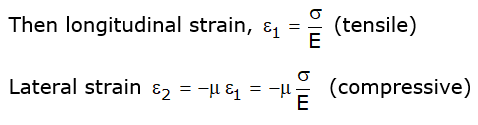
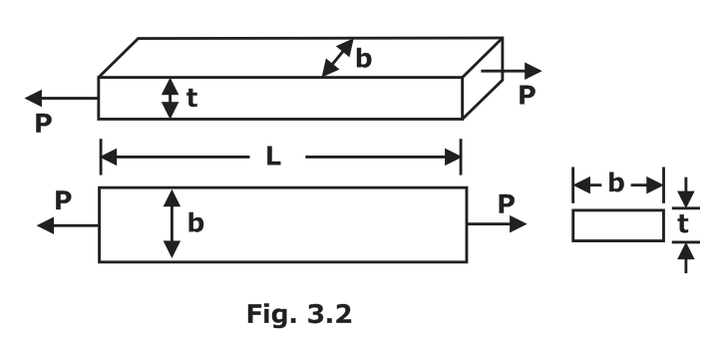
Fig. Volumetric strain
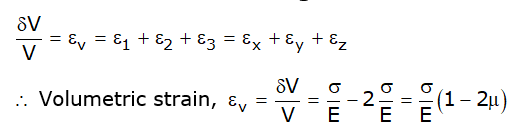
SHEAR MODULUS OR MODULUS OF RIGIDITY
The shear modules or modulus of rigidity expresses the relation between shear stress and shear strain.
![]()
where G = modulus of rigidity
ɸ = Shear strain (in radians) (also sometimes denoted by the symbol γ)
BULK MODULUS
When a body is subjected to three mutually perpendicular like stresses of equal intensity (σ).
Then the ratio of direct stress (σ) to the corresponding volumetric strain (ϵv) is defined as the bulk modulus K for the material of the body.
Which is generally denoted as ‘K’

RELATION BETWEEN DIFFERENT ELASTIC PROPERTIES
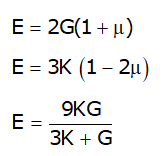
Value of any Elastic constant should be ≥ 0
E, K, G > 0
µ ≥ 0 [µcork = 0]
If K should be positive,
Then 1 – 2µ ≥ 0
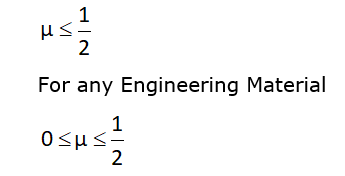
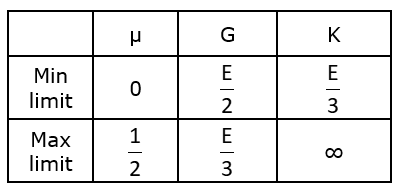
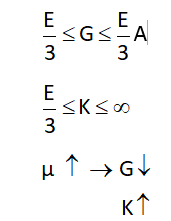
Always
G ≤ E
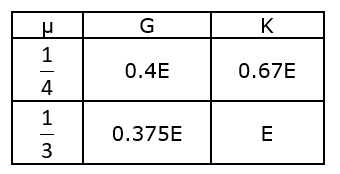
For metals
No comments:
Post a Comment
Knowing brings controversy